How To Find The Slope Of A Geometric Sequence
Understanding the intricacies of geometric sequences is essential for students and enthusiasts alike. These sequences are not just mathematical curiosities; they have real-world applications in fields like finance, science, and engineering. Just as a line has a slope that indicates its steepness, geometric sequences have a characteristic that reflects their growth rate. Learning how to find the slope of a geometric sequence can open doors to a deeper understanding of mathematics and its practical implications.
In this guide, we will explore the concept of a geometric sequence, its properties, and the methods you can use to find its slope. By the end of this article, you will not only understand the theoretical foundations but also be able to apply them practically. Whether you’re a student preparing for an exam, a teacher looking for ways to explain the concept, or just a curious mind, this article caters to all.
As we delve into the world of geometric sequences, we will answer crucial questions that will guide your learning journey. How to find the slope of a geometric sequence? What tools do you need? And how can you apply this knowledge in real-world scenarios? Let’s find out!
What is a Geometric Sequence?
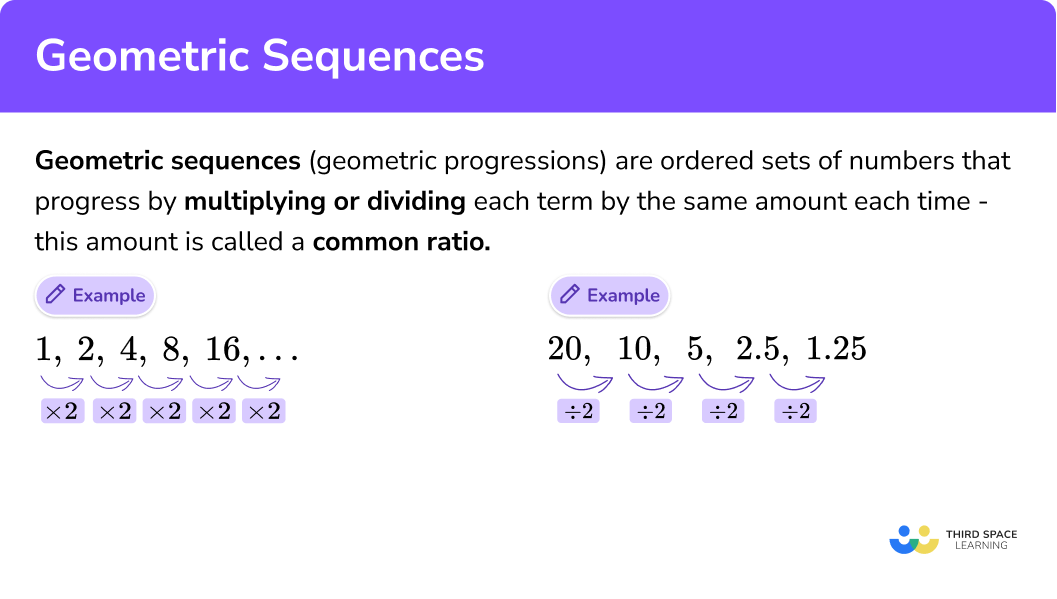
A geometric sequence is a sequence of numbers where each term after the first is found by multiplying the previous term by a fixed, non-zero number called the common ratio. For example, in the sequence 2, 6, 18, 54, the common ratio is 3. Geometric sequences can grow or decay exponentially, making them fascinating to study.
How to Identify the Common Ratio?
Identifying the common ratio is the first step in understanding how to find the slope of a geometric sequence. To find it, divide any term in the sequence by its preceding term. For example, if you have the sequence 4, 12, 36:
- Common ratio = 12 / 4 = 3
- Common ratio = 36 / 12 = 3
In both cases, the common ratio is consistently 3.
How to Find the Slope of a Geometric Sequence?
Finding the slope of a geometric sequence involves understanding how the terms of the sequence grow relative to each other. In a geometric sequence, the slope can be thought of as the rate of change between the terms. To calculate it, you can use the formula:
Slope = (a_n - a_(n-1)) / (n - (n-1))
Where a_n is the nth term and a_(n-1) is the previous term.
Why is Slope Important in a Geometric Sequence?
The slope in a geometric sequence indicates how rapidly the sequence is increasing or decreasing, making it crucial for applications such as calculating compound interest or understanding population growth. A steeper slope signifies a rapid increase, while a gentler slope indicates a slower growth rate.
Can You Visualize the Slope of a Geometric Sequence?
Yes! Visualizing the slope of a geometric sequence can enhance your understanding. You can plot the terms of the sequence on a graph where the x-axis represents the term number and the y-axis represents the term value. The line connecting these points will help you see the steepness of the slope. The steeper the line, the greater the slope.
How to Apply the Slope of a Geometric Sequence in Real Life?
Understanding how to find the slope of a geometric sequence can be applied in various fields:
- Finance: Calculating compound interest.
- Biology: Understanding population growth rates.
- Physics: Analyzing exponential decay in radioactive substances.
What Are the Limitations of Slope in Geometric Sequences?
While understanding how to find the slope of a geometric sequence is valuable, it also has its limitations. For instance, a geometric sequence assumes a constant growth rate, which may not always be the case in real-world scenarios. Additionally, the concept of slope is less applicable to non-linear sequences.
Conclusion: Mastering the Slope of a Geometric Sequence
In conclusion, mastering how to find the slope of a geometric sequence is not just about solving equations; it's about grasping the dynamics of exponential growth and decay. By understanding the common ratio, calculating the slope, and visualizing it, you'll be equipped to tackle real-world problems. Whether you're a student, a teacher, or an enthusiast, the knowledge of geometric sequences will serve you well in your mathematical journey.
You Also Like
Unveiling The Enigma Of Loona X Octavia: A Unique Fusion Of PersonalitiesUnveiling The Art Of Brave Casting: A Journey Into The Spotlight
Discovering The Allure Of Walmart Rings
Unlocking The Magic Of Disney Collab: A Journey Through Creativity And Imagination
Unpacking The Meaning Behind "So Fetch": A Cultural Phenomenon
Article Recommendations
ncG1vNJzZmiZlKK2r3rBqKmdnaKhrq%2Bw0mespGaTpLpwwNGynJygn2l8qbvWZquoZZaeu6V506GcZqucpL2mec6fZJpll5q8rrHTq6CcZaOavraxzZycZ6Ckork%3D